Dimensional Analysis

量纲分析

Some of the lectures will be accompanied byspecial lessons on Unit and Dimensional Analysis. While these lessons are notessential for following the video lectures, they will increase yourunderstanding of the physics and they will give you a different perspective onequations and formulas.

一些讲座将附有单元和量纲分析的特别课程。虽然这些课对于视频讲座来说不是必要的，但它们会提高你对物理的理解，而且它们会给你对方程和公式的不同看法。

What makes an equation?

方程式是什么？

In school one often thinks about equationsas formulas. The aim of a formula is to shorten or condense information into anexpression so that you don't need to worry about the derivation. You want toknow the volume of a sphere, you look for the right formula (V=4πr³/3),you plug in the value for the radius, you plug in the value for π, and you aredone.

在学校里，人们常常把方程看成公式。公式的目的是将信息缩短或浓缩到表达式中，这样就不必担心派生问题。你想知道一个球体的体积，你找到正确的公式（V = 4πR³/ 3），你插入值为半径，你带入π的值，你就得出了结果。

The purpose of a formula is that you don'tneed to worry about it. Once you know what formula to use things are easy and you can worry about a different part of the problem.

公式的目的是你不必担心它。一旦你知道使用什么样的公式是容易的，你就会担心问题的不同方面。

Equations (as we will look at them in thiscourse) are different. Equations are something you want to contemplate and tounderstand how they were derived. Their fundamental role is not to shorten yourcomputations, but to express a relation, to equate two physical quantities. Theeasiest and most accessible way of contemplating an equation is to think aboutits dimensions.

Units and Dimensions

方程式（我们将要在这门课中学）是不同的。方程是你想思考并理解它们是如何派生出来的。它们的基本作用不是缩短计算，而是表达一个关系，使两个物理量相等。考虑方程的最简单、最容易获得的方法是考虑它的单位和维度

You are all familiar with physical units.You know that a length can be measured in meters, miles, inches, kilometersetc; a time can be measured in seconds, hours, minutes etc; or a velocity canbe measured in miles per hour, m/s, km/h etc.

你们都熟悉物理单位。你知道一个长度可以用米，英里，英寸，公里等来测量；一个时间可以用秒，小时，分钟等来测量；或者一个速度可以用英里、小时、米、公里等来测量。

Whenever a physical quantity is measured inunits of length we will say that its dimension is a length, and we will denotethat by L; whenever a quantity is measured in units of time, we will say itsdimension is time, and we will denote that by T.

每当一个物理量用单位长度来度量时，我们会说它的维数是一个长度，我们用L表示；当一个量用时间单位来度量时，我们会说它的维数是时间，我们将用T表示。

The dimension of velocity can be expressedin terms of the fundamental dimensions of Length and Time. We know that, bydefinition, a velocity is a length divided by time. We will express that bysaying that the dimensions of velocity are L/T.

速度的大小可以用长度和时间的基本维度来表示。我们知道，根据定义，速度是除以时间的长度。我们会说，速度的大小是L/T。

The purpose of dimensions is to do awaywith the clutter of numbers and units. Often, it is easy to forget that mph(miles per hour) and m are two fundamentally different units because they referto quantities which cannot be equated. A velocity will never be equal to alength; a volume cannot be equal to a length. The purpose of dimensions is tomake sure we are never comparing apples with oranges.

维度的目的是消除数字和单位的杂乱。通常，很容易忘记MPH(每小时/英里）和M是两个根本不同的单位，因为它们指的是不能被等同的数量。速度永远不会等于一个长度；一个体积不能等于一个长度。维度的目的是确保我们从不把苹果和橙子作比较。

The advantage of dimensions over units isthat it really forces us to think about fundamental quantities. For example, isa light-year comparable to a meter, to a second or to a meter per second? Toanswer that question you must know that a light-year is a measure of distance.In this course we will say that a light-year has dimensions of distance.

维度比单位的优势在于它确实迫使我们去考虑基本的数量。例如，一光年和一米，一秒或一米每秒，它们是如何比较的？要回答这个问题，你必须知道光年是距离的量度。在这个过程中，我们会说光年有距离的维度。

To keep things, clear we will use squarebrackets to denote the dimensions of a physical quantity. For example, if thevelocity of a car is v=10 miles/hour, the dimensions of this quantity will be:

为了保持事物清晰，我们将使用方括号来表示物理量的维度。例如，如果汽车的速度是v = 10英里/小时，这个数量将是：

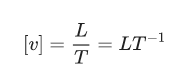
Notice that the numerical values do notaffect the dimensions of a quantity.

请注意，数值不影响数量的维数。

The Fundamental Rule of DimensionalAnalysis

量纲分析的基本规则

Dimensions on the left = Dimensions on theright

左边的维度=右边的维度

As we said, the purpose of dimensionalanalysis is to make sure we are always comparing apples with apples and orangeswith oranges. Let’s say you want to write down the distance d covered by somecar that moves with constant velocity v in the time Δt. If you haven’t taken aphysics course in some time you might not remember the actual formula, and youcould scramble different answers:

正如我们所说，量纲分析的目的是确保我们总是把苹果与苹果、橙子和橙子作比较。比方说，你要写下距离D被一些汽车移动以恒定速度v在时间ΔT.如果你没有采取物理课程在一些时候你可能不记得具体的公式，你可以争夺不同的答案：

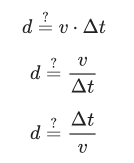
Dimensional Analysis solves the problem foryou! Let’s compare the dimensions for each of these equations:

量纲分析解决了你的问题！让我们比较每个方程的维数：

Plugging in the dimensions for thesequantities we get:

插入我们得到的这些量的大小：

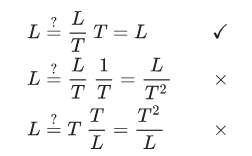
Dimensional Analysis tells us which equationis a possible candidate, and which equations can never be true.

量纲分析告诉我们哪种方程是可能的候选，哪些方程永远不可能成立。

The cool part about Dimensional Analysis isthat besides checking equations, you can also find equations (up to adimensionless constant, like 1/2 etc.). For example, let’s say we want todetermine all the possible equations we can find that relate a distance, anacceleration and time.

量纲分析的一个很酷的部分是，除了检查方程外，还可以找到方程（无量纲常数，如1/2等）。例如，我们想确定我们能找到的有关距离、加速度和时间的所有可能的方程。

The most general formula we can write down(again, ignoring any extra numerical factor) is this:

我们可以写的最一般的公式（再次忽略任何额外的数值因子）是：

x^m=a^pt^q

Where m,p,q are some rational numbers. Ifwe re-write this relation in terms of dimension we get:

其中m，p，q是有理数。如果我们用维数来写这个关系，我们得到

Matching the L and T terms imposes twoconditions m=p and q−2p=0. So the equation is now x^p=a^pt^2p.By taking a square root of p (the same way we can take a 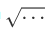on both sides of anequation), we are left with x=at². There is no other relation thatyou can write down that connects a distance, an acceleration and time.

匹配的L和T条件规定了两个条件M = P和Q−2P = 0。所以方程现在x^p=a^pt^2p。以P的平方根（我们可以取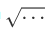在一个等式两边），留给我们的是x = at²。没有任何其他关系，你可以写下来，连接距离，加速度和时间。