Time Dilation

时间膨胀

Light-clock recap

光时钟概述             

We have used the light-clock as a thoughtmodel to illustrate the dilation of time in a moving frame, that is - anobserver at rest will see time passing slower in a moving frame. There are afew points that need to be clearly stated:

 我们用光时钟作为思维模型来说明运动框架中时间的膨胀，也就是说，静止的观察者在运动的框架中会看到时间过慢。有几点需要明确说明：

1.      There are two essential stepsin the argument about the light-clock:

1。关于光钟的争论有两个基本步骤：                                         

a. when the observer looks at the movingclock, they see light travelling over a longer distance to reach the othermirror

当观察者看到移动的时钟时，他们看到远处的光线到达另一面镜子。

b. light always travels at the same speed(Einstein's second principle of special relativity)

 光总是以同样的速度传播（爱因斯坦狭义相对论的第二原理）

Because time is distance over velocity, andthe distance has increased while the velocity has stayed the same, it meansthat the observer must conclude that light takes longer to reach the othermirror when the clock is moving (as compared to the clock at rest).

 因为时间是速度的距离，而距离是增加的，而速度保持不变，这意味着观察者必须得出结论：当时钟移动时（相对于静止的时钟），光需要更长的时间到达另一面镜子。

2.      Often when one encounters thelight-clock example for the first time, there is some confusion about the"mechanism" of the clock. Why would light move on a diagonal if youare moving the mirrors?

2。通常，当人们第一次遇到光时钟的例子时，对时钟的“机制”有一些混淆。如果你移动镜子，为什么光线会在对角线上移动？

To better visualize the setup think aboutthe light-clock as always sending light from dot A to dot B. When the clock ismoving (with constant, uniform velocity) the light ray still needs to travelfrom dot A to dot B, because nothing in the way the light-clock functions canchange. If the light ray wouldn't reach the dot B, then the principle of relativitywould be contradicted: because for an observer at rest the light on hislight-clock always moves from A to B, while for a moving observer the light onhis light-clock would go somewhere else, so he would know he is moving.

为了更好地可视化设置，当时钟移动（恒定不变的速度）时，考虑从A点到B点的光时钟，光线仍然需要从点A移动到点B，因为光时钟函数的任何方式都不能改变。如果光线不能到达B点，那么相对论的原理将是矛盾的：因为一个观察者在休息时，他的光钟上的光总是从A移动到B，而对于一个移动观察者，他的光钟上的光会转到别的地方，所以他会知道他在移动。

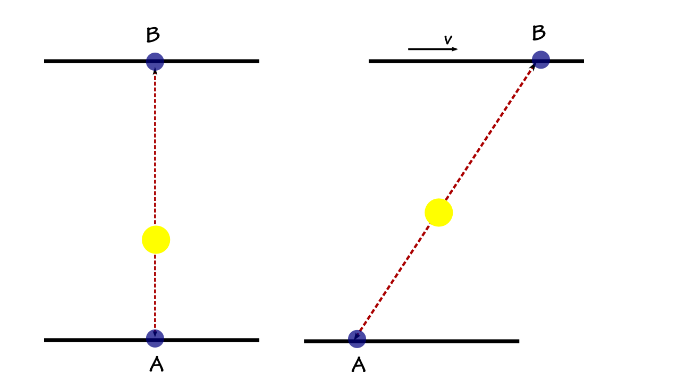
Alternatively, you could keep thelight-clock at rest, and move the observer. Nothing should change in ourreasoning. Now the moving observer will see light travelling on a diagonal pathbecause he sees everything moving in the opposite direction of his motion.

或者，你可以让光时钟静止，移动观测者。我们的推理没有什么变化。现在移动观察者将看到光线沿着对角线行进，因为他看到所有的东西都在他运动的相反方向移动。

3.      It is important to notice thatwhat matters is not the motion of the clock per se (remember there is noabsolute motion in relativity). It is only the relative motion between theobserver and the clock.

3.重要的是要注意，重要的不是时钟本身的运动（记住相对论中没有绝对运动）。它只是观察者和时钟之间的相对运动。

4. Even though we have used the light-clockto derive our result, the dilation of time applies to all inertial movingframes, and it is not restricted only to light-clocks or clocks moving withvelocities close to the speed of light. It wouldn't make much sense if twoclocks (a light-clock and a mechanical clock) would show the same time whenthey are at rest, but different times when moving with the same velocity. Ifthis were to happen, it would directly contradict Einstein's first principle ofrelativity (because if you were to notice a difference between your mechanicalclock and your light-clock, you could infer that you are in a moving frame).Have a look at the following Brain Teaser for a more detailed discussion ofthis.

4。虽然我们用光时钟来推导我们的结果，但时间的膨胀却适用于所有惯性运动的框架，它并不局限于以接近于光的速度运动的光钟或钟。如果两个时钟（一个轻时钟和一个机械时钟）在静止时显示相同的时间，但在同一速度下移动的时间不同，那就没有多大意义了。如果发生这种情况，它将直接与爱因斯坦的第一相对论相悖（因为如果你注意到你的机械钟和你的光钟的区别，你就可以推断出你处于移动的框架中）。请看下面的关于这个问题的更详细的讨论。

The Amount of Time Dilation

 时间膨胀量

The light-clock is a great example not onlybecause it allows us to understand the dilation of time, but it also gives us away of easily deriving the amount with which time dilates. If you want to seethe mathematical derivation, check the supplement in the next unit. Thederivation is very basic and it only uses algebra and Pythagoras' theorem.

光时钟是一个极好的例子，因为它不仅可以让我们了解时间的扩张，但它也给我们提供了一种容易获得，时间使量。如果你想看数学推导，检查下一单元的补充。推导是很基本的，它仅使用代数和毕达哥拉斯定理。

What we find from the light-clockderivation is this:

我们从光时钟的推导中发现的是：             

if an observer at rest compares the timekept by his rest clock with the time that he sees on a moving clock, he willconclude:

如果一个静止的观察者将他的休息时间与他在一个移动时钟上看到的时间进行比较，他就会得出结论：

1.      Qualitative conclusion: onesecond on the moving clock takes more than a second on the rest clock, as seenby the rest observer

1。定性结论：在移动时钟上的一秒钟需要在休息时间超过1秒，如其他观察者所见。             

2. Quantitative conclusion: one second onthe moving clock takes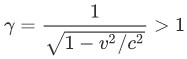seconds on the rest clock, as seen by the rest observer

( γ>1 for v>0 - see the derivation inthe exercise below).

 2。定量的结论：上一秒钟移动的时钟以γ= 11−V2／C2＞1秒的休息时钟，通过其他的观察者              （γ＞1为了v＞0）参见下面练习中的推导）。

To visualize what we mean by this, imaginethat the rest observer uses light-clocks and that it takes 1 second for lightto travel from the lower mirror to the upper mirror. Then, if the two light-clocksare synchronized such that the light rays leave the bottom mirror at the sametime, then when light reaches the upper mirror on the rest clock, it onlytraveled part of the distance on the moving clock. And when it reaches theupper mirror on the moving clock, the light on the rest clock is already movingtowards the bottom mirror.

想象一下我们的意思，想象一下，其余的观测者使用光时钟，光从下反射镜到上反射镜需要1秒的时间。然后，如果两个光时钟同步，使光线离开底部镜子在同一时间，然后当光到达上镜子的休息时钟，它只走一段距离的移动时钟。当它到达移动时钟的上反射镜时，静止时钟上的光已经向底部反射镜移动。

The relation between the amounts of time isthis:

时间量之间的关系是这样的：

a.      when 1 second has passed on therest clock, 1/γ seconds have passed on the moving clock, as seen by the restobserver

当1秒传递到静止时钟时，1秒/γ秒已经传递到移动时钟上，如其他观察者所见。                           

b.      when 1 second has passed on themoving clock, γ seconds have passed on the rest clock, as seen by the restobserver

当1秒传递到移动时钟时，其余的观察者看到了秒数。

If you find γ confusing, you can replace itby some arbitrary value, let's say 2 (you can easily compute what velocity thisactually corresponds to):

如果你发现γ混淆，你可以用任意值替换它，比如说2（你可以很容易地计算出这个对应的速度）：

a.      1 second on rest clockcorresponds to 0.5 seconds on moving clock, as seen by the rest observer

在休息时钟上的1秒对应于移动时钟的0.5秒，如其他观察者所看到的。             

b.      1 second on the moving clockcorresponds to 2 seconds on the rest clock, as seen by the rest observer

在移动时钟上的1秒对应于静止时钟上的2秒，如其他观察者所看到的。

We always say for the observer at rest,because if there was a second observer moving with the moving clock, he wouldsee everything the other way around: for the second observer the moving clockwould be at rest (because he is moving with it), and the rest clock would beslowed down (because it's moving with respect to him).

我们总是说观察者在休息，因为如果有第二个观察员移动时钟，他会看到一切的另一方面：第二个观察员的移动时钟将休息（因为他正在移动），其余的时钟将放慢（因为它的移动与他）。

If you replace the clocks by twins, youwill get the famous Twin Paradox (which we will cover in greater detail in afuture lesson): each twin sees their moving sibling as being younger.

如果你用双胞胎取代时钟，你将得到著名的孪生佯谬（我们将在以后的课程中详细介绍）：每个双胞胎都认为他们的兄弟姐妹更年轻。

The Gamma Factor

γ因子             

In a way, you can think about γ factor as aconversion coefficient between two units. E.g. 1 meter=3.28 feet. Thedifference is that we are actually talking about seconds in both cases, butmeasured in different frames of reference. When the clocks stop moving we recover1 second= 1 second.

在某种程度上，你可以把γ因子看成是两个单位之间的转换系数。1米＝3.28英尺。不同的是，我们实际上是在两种情况下谈论秒，但在不同的参照系中测量。当时钟停止移动时，我们恢复1秒= 1秒。

Let's condense our discussion in a formula.An observer at rest wants to know how much is an interval of time Δt on hisrest clock versus a moving clock. Then he will write:

让我们把讨论浓缩成一个公式。一个静止的观察者想知道时间ΔT在他休息的时钟与时钟移动间隔是多少。然后他会写：                  

(See next unit for the derivation of γ).

（参见下一个推导γ的单位）。

Note: By interval of time we mean theamount of time between two events. Both the rest frame of reference and themoving frame of reference agree that the two events take place, but they won'tagree about the difference in time between them. However, they will agree thatthe ratio between the two intervals of time is given by γ.

注意：时间间隔是指两个事件之间的时间量。其余的参照系和移动参照系都同意这两件事发生了，但他们对时间的不同意见不一致。然而，他们会同意两个时间间隔的比值是由γ给出的。

Note: These are the only two relations thatyou need to know for this lesson and the quiz. However, to do well on the quizit is essential to understand the meaning behind the formula. When you approachthe quiz don't think about how to plug the numbers into the formula, but how tomake physical sense of the problem. Visualize each problem and try to expressit in your own words.

注意：这是本课和测验中你需要知道的仅有的两个关系。然而，要想在测验中取得好成绩，理解公式背后的含义是很重要的。当你接近测验时，不要考虑如何把数字插入公式中，而是如何使问题有物理意义。想象每一个问题，并试着用你自己的语言表达出来。

The aim of the quiz is not to make you proficientat solving this type of problems, but it should give you the ability ofchecking your final answers. You should be able to know if your answer makessense or not.

测验的目的不是让你熟练地解决这类问题，而是让你有能力检查你的最终答案。你应该能够知道你的回答是否有道理。

Example

例子

Imagine that an observer at rest is lookingat his rest clock and at a clock moving with a velocity of v=0.5 c. We willoften express velocities in terms of c, the speed of light, to ease yourcomputations. If the observer sees 1 second passing on his rest clock, how manyseconds would he see that have passed on the moving clock?

想象一个静止的观察者正在观察他的休息时钟和一个以v＝0.5 C的速度移动的时钟。我们通常用C，即光的速度来表示速度，以简化计算。如果观察者看到他的休息时钟经过了1秒，他看到移动时钟经过了多少秒？

We start by finding γ for the givenvelocity:

我们从寻找给定速度的γ开始：

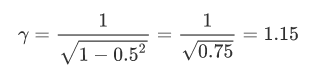
You can plug in this result in the aboveformula, or you can simply reason about it directly. If 1 second on the movingclock, is gamma seconds in the rest clock, how many seconds on the moving clockis 1 second on the rest clock (as seen by rest observer)?

你可以在上面的公式中插入这个结果，或者你可以直接解释它。如果移动时钟上的1秒是静止时钟中的伽玛秒，那么移动时钟上的秒数是静止时钟上的1秒（休息观察者所见）？

Our result is less than one second as weexpected. Always check your intuition!

我们的结果比我们预期的少了一秒。请永远检查你的直觉！             

·The γ factor, 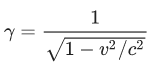, might seem very off-putting, so let'sbuild some intuition about it. The goal is to understand how γ depends on v.There are a few special cases to consider:

γ因子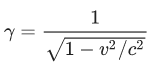，，看起来很令人讨厌，所以让我们建立一些关于它的直觉的知识。我们的目标是了解γ如何依赖于V：

In the last case, v>c, γ does not exist(it does not have a real solution) because v²/c²>1 and1−v²/c²<0 and we cannot take the square root of anegative number without obtaining an imaginary part. The physicalinterpretation is that nothing can move faster than the speed of light. And ifa reference frame could move with the speed of light, everything would seemfrozen in time (nothing in such a frame could move, because then it would haveto move faster than light).

在最后一种情况下，V＞C，γ不存在（它没有真正解决）因为v²/c²>1和1−v²/c²<0，我们不能没有获得一个虚构的部分以一个负数的平方根的方式。物理解释是，没有什么东西能比光的速度运动得快。如果一个参考帧能随着光的速度移动，那么所有的东西都会在时间上被冻结（这样的帧中没有任何东西可以移动，因为它必须比光运动得更快）。