Unit Analysis

单位分析

We are used from Newtonian mechanics tothink about distances, time intervals and velocities in terms of units likemph, m, km, s, m/s etc. However, in special relativity the scale where therelativistic phenomena become visible is very different. Because this scale isdetermined by the speed of light, it would greatly simplify our calculationsand reasoning if we were to employ a system of units adapted to this constant.

我们用牛顿力学来考虑距离、时间间隔和速度，例如单位英里数、米、公里、秒、米/秒等。然而，在狭义相对论中，相对论现象可见的尺度是非常不同的。因为这个尺度是由光的速度决定的，如果我们采用一个适应这个常数的单位系统，它将大大简化我们的计算和推理。

Distances

距离

The speed of light in the vacuum isapproximately

真空中光的速度大约是

c≈300 000 000 m/s

This is an inconveniently long number. Weeither need a larger unit for distance, or a smaller unit of time. For manyreasons, the second seems to be a more adequate unit of time. Instead, we canintroduce a new unit of distance, defined as the distance covered by light inone second.

这是一个大数目。我们需要一个更大的距离单位，或者更小的时间单位。由于许多原因，第二个似乎是一个更适当的时间单位。相反，我们可以引入一个新的距离单位，定义为光在一秒钟内所覆盖的距离。

 We'll call this the light−second:

我们叫这光秒：

1 light−second=1 c⋅s≈300 000 000 m

Notice that the light-second is a unit fora dimension of distance.

请注意，光秒是距离维度的单位。

Using our new unit, we can re-write c as:

使用我们的新单元，我们可以重写C作为：

c=1 light−second/second

Velocities

速度                          

It is a consequence of special relativitythat there are no velocities greater than the speed of light. This makes thespeed of light into a natural scale for measuring velocities. You have alreadyseen that γ was defined in terms of v/c. This suggests that it would be auseful simplification to express all velocities in units of c. For example,v=0.1 c instead of writing v=30 000 000 m/s.

狭义相对论的结果是，没有比光的速度更大的速度。这使得光的速度成为自然的尺度来测量速度。你已经看到，γ是用V／C定义的，这表明用C单位表示所有速度是很有用的简化，例如，v＝0.1 C，而不是写V＝30 000 000 m／s。

Then we would re-write γ as:

然后我们再写γ：

where v is measured in units of c.

其中v是用C单位测量的。                           

The utility of these units will become muchclearer in Lecture 4.

这些单元的效用将在第4课中变得更加清楚。            

Natural Units

自然单位

In our previous discussion of dimensionlessanalysis, we have tried to motivate the choice of time and distance asfundamental dimensions. At the same time, because the speed of light is auniversal constant, it could suggest us that we can also express all thedistances in terms of the speed of light and some time unit. For example, ameter could be defined as

在我们以前关于无量纲分析的讨论中，我们试图把时间和距离的选择作为基本维度。同时，因为光的速度是一个宇宙常数，它可以暗示我们也可以用光的速度和一些时间单位来表示所有的距离。例如，米可以定义为

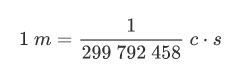
So now our two yardsticks are c and s. Butthere is a fundamental difference between them: c has a well defined physicalmeaning, while s still needs to be defined in terms of some arbitrary numericalvalue. A second is defined as the "the duration of 9 192 631 770 periodsof the radiation corresponding to the transition between the two hyperfinelevels of the ground state of the caesium 133 atom" (SI Brochure, Section2.1.1.3). Because we wanted to preserve the historical value of the second, wehad to introduce that messy number.

所以现在我们两个尺度是C，但他们之间有着根本的区别：C有明确的物理意义，同时还需要在一些任意的数值来定义。第二个定义为“时间的9 192 631，对应的辐射的770个周期的过渡之间的两个超精细能级的基态铯133原子”（SI Brochure，第2.1.1.3）。因为我们想保留第二个的历史价值，所以我们不得不引入这个混乱的数字。

Because the speed of light is free of anyarbitrary values, it's called a natural unit. You should be careful, becausewhen using natural units some physicists like to write c=1, but this is not areal equality, but a misnomer. c is a dimensionful quantity ([c]=L/T) while 1is dimensionless.

因为光的速度没有任何任意的值，所以它被称为自然单位。你可要小心了，因为当采用自然单位一些物理学家喜欢写C = 1，但这不是真正的平等，但用词不当。C是一个dimensionful数量（[C] = L / T），1是无量纲。             

We will continue our discussion of naturalunits in the future lessons. Our goal is to show how we can employ otherfundamental constants to develop a system of natural units.

在以后的课程中，我们将继续讨论自然单元。我们的目标是展示我们如何使用其他基本常数来开发自然单位系统。