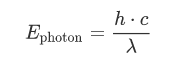
Physics Primer: Classical waves  

物理学入门：古典波

Review of Classical waves

经典波述评             

In classical physics, a wave is describedby three quantities: wavelength, frequency and amplitude. The wavelength, λ(lambda), is the distance between two consecutive crests, while the amplitudedetermines the height of the wave. The period, T, of a wave is the amount oftime after which the height of the wave at a particular point in space returnsto its initial value. You can see these quantities in the figure below, inwhich we have represented the same wave at three different moments in time: 0,T/2, T. The frequency, ν (nu), is the inverse of the period T: ν=1/T. 

在经典物理学中，一个波由三个量来描述：波长、频率和振幅。波长（λ），是λ连续两个波峰之间的距离，而振幅决定波的高度。波的周期t是波在空间某一点的高度回到其初始值的时间。你可以在下面的图中看到的这些数量，在我们代表相同的波在三个不同的时间的时刻：0，T/2，T的频率，ν (nu)，是这一时期的逆：ν=1/T    

A word of caution: ν and v are easilymistaken. The former is a frequency, while the second is a velocity. If youhave problems distinguishing between the two you can always consider theirdimensions:

 提醒一句：ν和V的易犯错误。前者是频率，第二个是速度。如果你有区分两者的问题，你可以考虑它们的单位：

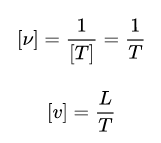
Because ν and v have different dimensionsyou should always be able to distinguish between the two in an equation.

因为ν和V有不同的维度，你都应该能够区分两方程。                          

The velocity of a wave

波的速度

The velocity of a wave is the product ofits wavelength and frequency. You can see the intuition behind this looking atthe above image: a point on the wave moves over a distance λ in a time T. Thismeans that the wave velocity u could be written as:

波的速度是它的波长和频率的乘积。你可以看到这背后看着上面的图像的直觉：一点的波动在时间t这意味着波速度u可以写成一个距离λ：

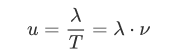
In the case of light, its speed c in thevacuum is a constant, so if we know the wavelength of a light wave we also knowits frequency, and if we know its frequency we know its wavelength.

在光的情况下，它在真空中的速度c是常数，所以如果我们知道光波的波长，我们也知道它的频率，如果我们知道它的频率，我们知道它的波长。             

A word of caution: Though one commonlyspeaks about bright colors, we'll only use brightness to refer to the intensityof light, but this has no connection with the color of this light. Color willbe solely determined by the wavelength or frequency of light. This is asimplification because most real-life light contains a spectrum of wavelengths.Sun light (white light) contains all the wavelengths of the visible spectrum.We are only going to use monochromatic light waves, i.e. waves with a uniquewavelength (or frequency).

注意一点：虽然人们通常谈论明亮的颜色，但我们只使用亮度来表示光的强度，但这与光的颜色没有关系。颜色完全取决于光的波长或频率。这是一个简化，因为大多数现实生活中的光包含一个波长的光谱。太阳光（白光）包含可见光谱的所有波长。我们只使用单色光波，即具有独特波长（或频率）的波。

As you could easily guess, the amplitude ofa wave influences the energy of that wave. The higher the amplitude, the higherthe energy. Think about water waves: the higher the waves, the greater thequantity of water and thus also the amount of energy necessary to generate sucha wave. At least, this is our intuition about mechanical waves, but as Einsteinhas shown things are radically different for light (i.e. electromagneticwaves).

正如你所能猜到的，波的振幅影响波的能量。振幅越大，能量越高。想想水波：波浪越高，水的数量就越大，产生波浪的能量也就越大。至少，这是我们对机械波的直觉，但是正如爱因斯坦所展示的，光的本质是不同的（即电磁波）。             

Units of wavelength and frequency

波长和频率单位

Wavelengths have dimensions of length. Thewavelengths associated with light are extremely small, of the order of abillionth of a meter, or 10⁻⁹ m. The unit corresponding to thisvalue is called a nanometer or nm:

波长有长度的长度。与光的波长非常小，一米第十亿的订单，或10⁻⁹ m单位对应于这个值被称为纳米或纳米：

1 nm=10⁻⁹ m

Because frequency is the inverse of aperiod (or oscillations per second), it has dimensions of T⁻¹. Theunit associated with this dimension is s⁻¹ which is also called aHertz or Hz:

由于频率是一个时期的逆（或振荡每秒），它有1个维度的 T⁻¹。与此相关的单元维度的s⁻¹也叫赫兹Hz或：

1 Hz=1 s⁻¹

The frequency of light is very high (manymany oscillations per second), of the order of 10¹⁴ Hz.

光的频率非常高（每秒有许多振荡），频率为10¹⁴赫兹。                          

Problem

Quantum Waves

量子波             

The intensity of a wave is proportionalwith the square of the amplitude, and for mechanical waves it's alsoproportional with the energy of the wave. However, this is different in thecase of light.

波的强度与振幅的平方成正比，对于机械波，它也与波的能量成比例。然而，在光的情况下，这是不同的。

In his 1905 article "Concerning anHeuristic Point of View Toward the Emission and Transformation of Light",Einstein extended Planck's equation to claim that the energy of a ray of light(or of the photons associated with it) only depends on its frequency:

在他的1905篇文章“关于光的发射和转化的启发式观点”中，爱因斯坦扩展了普朗克的公式，声称光线（或与之相关的光子）的能量只取决于它的频率：

Alternatively, if you use c=λν, you canwrite:

另外，如果你使用C =λν，你可以写：